Stopping Distance (GCSE Physics)
Stopping Distance
Calculating Stopping Distance
The stopping distance of a vehicle is the distance it takes for a moving vehicle to come to a complete stop, which can be calculated by adding together the thinking and braking distances:
Thinking Distance
The thinking distance is the distance travelled in the thinking time it takes to think and react to a hazard on the road. It is the time between the driver seeing the hazard and them hitting the breaks.
Thinking distance can be affected by several factors:
- Human variation – for humans, normal reaction time can be anywhere between 0.2 to 0.9 seconds. This is the time it takes for a driver to react to a stimulus on the road and actually press the brakes. From person to person, reaction times can vary drastically.
- External influences – Thinking time can also be affected heavily by alcohol, drugs, distractions and tiredness. This is one of the big risks of ‘drink driving’ – having slow reactions.
Braking Distance
The braking distance is the distance travelled between the moment the driver hits the breaks to the moment the car.
When a driver sees a hazard, such as a child running into the road, they may stop suddenly. This is called an emergency stop. Once the driver presses on the brakes, it still takes the vehicle some time to stop. This is the braking distance.
Braking distance can be affected by several factors including:
- Force of breaking – the force of braking will affect the distance it takes for the car to brake. Normally most drivers would push down fully on the breaks, so this is less important for an emergency stop
- Speed of the vehicle – the faster a vehicle is travelling, the greater the braking force needed to stop the car. This means that the braking distance will also increase, meaning that the total stopping distance will also increase.
- Mass of the vehicle – if the vehicle has a very low mass, then it will travel further along a surface, resulting in a larger braking distance
- Road conditions – if the road surface is smooth (for example when it is icy or rainy) then the vehicle will travel further along the surface, resulting in a larger braking distance.
Example Scenarios
Question: This diagram shows the thinking and braking distance of a car driver when he spots a junction coming up and breaks at full power. What would happen to thinking and braking distance if a) the driver was under the influence of alcohol, b) the driver was travelling at a faster speed.
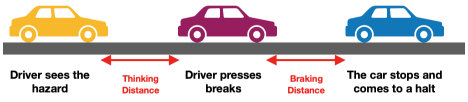
a) Alcohol: If the driver was under the influence of alcohol, his reactions would be slower, so thinking distance is higher. We assume speed is constant and the car is working normal, so braking distance is unchanged.

b) Higher Speed: If the car was travelling faster, it would take longer for it to stop once the brakes are pressed. This means that braking distance rises. In addition, thinking distance will increase. Even though the driver’s reactions may not be compromised and his thinking time could stay the same, he will have travelled more in that same thinking time due to his high speed.
Braking Distance
As an object (such as a car) brakes and comes to a halt, work is done to remove the kinetic energy. This means that the work done equals the kinetic energy. Therefore we can form an equation to calculate the braking distance.
Forming the Equation
1. Write the equation to calculate kinetic energy.
E k = ( m v ² ) / 2
2. Write the equation to calculate work done.
W = f x d
3. Kinetic energy equals work done.
E k = W
4. Substitute in your two equations.
( m v ² ) / 2 = f x d
Calculating the Braking Distance

Where:
- mass, m, in kilograms, kg
- speed, v, in metres per second, m/s
- force, f, in Newtons, N
- distance, d, in metres, m
Example
Question: A car has a mass of 850kg and moves at a speed of 10m/s. What will the braking distance of the car be, if it has a breaking force of 1800N? Give your answer to 3sf.
1. Write the equation to calculate the braking distance.
( m v ²) / 2 = f x d
2. Substitute in the numbers.
( m v ² ) / 2 = f x d
( 850 x 10² ) / 2 = 1800 x d
3. Find the braking distance.
Solve for d to find the braking distance.
( 850 x 100 ) / 2 = 1800 x d
85000 / 2 = 1800 x d
42500 = 1800 x d
d = 42500/1800
d = 23.6111…
d = 23.6m (3sf)
Graphs for Stopping Distance
- The thinking distance will increase linearly as the speed increases.
- The braking distance will increase non-linearly as the speed increases. The faster a vehicle is travelling, the longer the braking distance will be.
The main thing that you need to remember is that as the speed of a vehicle increases, the total stopping distance will increase.
Question: A traffic analyst is scrutinising some data on three crashes that have occurred in his country district. He is looking at 3 independent crashes here, and looking at a single vehicle from each crash. He needs to make an early prediction on whether i) any of the drivers were under the influence of alcohol, ii) the road conditions affected the stopping distance. He has asked you for your opinion. Explain your observations from the graph below.
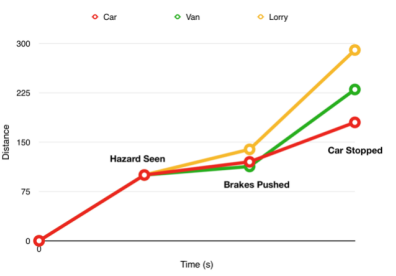
This graph is quite kind to us, as it breaks down the different stages quite nicely. You can easily spot the thinking distance and braking distance. Let us consider each one
- The thinking distances are all quite similar for the cars. They all rise a similar amount. You might be tempted to speculate that the lorry driver was under the influence of alcohol, and further investigation would be useful, but realistically the thinking distances are quite similar between all 3 vehicles. Remember, there is intra-person variation which happens due to differences in reactions from person to person.
- The braking distances are quite different for the cars. You might be tempted to speculate that the lorry was driving on an icy road, which could explain an increased braking distance. However, you have to remember that each vehicle has a different mass. The lorry must be very heavy, and this explains its larger braking distance.
Overall, the results are what you would expect, so it is unlikely that alcohol or poor road conditions were influencing factors in these crashes.
Stopping distance is the total distance that an object travels from the time when its brakes are applied to the time when it comes to a complete stop. It is a measure of the time and distance required for an object in motion to stop.
To calculate stopping distance in physics, you need to know the speed of an object, the reaction time of the driver, and the braking distance of the vehicle. You can use the formula stopping distance = reaction distance + braking distance to calculate the stopping distance.
Reaction distance is the distance that an object travels from the time when a driver first sees a danger to the time when they apply the brakes. It is a measure of the driver’s reaction time.
Braking distance is the distance that an object travels from the time when the brakes are applied to the time when it comes to a complete stop. It is a measure of the effectiveness of the brakes and the friction between the tires and the road.
Speed has a major impact on stopping distance in physics. The faster an object is moving, the greater its stopping distance will be. This is because the braking distance of a vehicle increases as its speed increases, and so does the reaction distance of the driver.
Stopping distance is an important concept in physics that has many real-world applications. It is used in transportation to design and maintain vehicles and roadways, to evaluate the safety of vehicles and roads, and to develop traffic control systems. It is also used in sports to measure performance and in many other areas where the motion of objects needs to be measured and analyzed.
Yes, you can reduce stopping distance by driving slower. This is because the braking distance of a vehicle decreases as its speed decreases, and so does the reaction distance of the driver. Driving slower can also help you to react more quickly and effectively to road hazards, making it safer for you and other drivers on the road.





Still got a question? Leave a comment
Leave a comment