Practice Model of Water - 3.1.1 Density of Materials (GCSE Physics AQA)

Density of Materials

Calculating Density

Density is a measure of how compact a substance is. It is a measure of how heavy an object is for its volume. We can calculate density using the following formula:

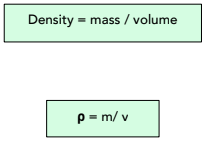
Where:

• density, ⍴, in kilograms per metre cubed, kg/m³
• mass, m, in kilograms, kg
• volume, V, in metres cubed, m³

The most common units for density are g/cm³, followed by kg/m³. A density of 1 g/cm³ means each cm³ of the substance weight 1g.

Question: Finola has a brick with volume 146cm³ and mass 4,500g. Calculate the density of the brick with correct units, to 1 dp.

1. Write out the equation.

⍴ = m/ v

2. Convert the units. In the question, we have been given the mass in grams, but to use the equation we need a mass in kilograms. Similarly, we have been given the volume in cm³, so we need to convert into m³.

Since 1000g = 1 kg
4500 grams = 4.5 kg
Since 1,000,000 cm³ = 1m³
146 cm³ = 0.000146 m³

3. Substitute in the numbers.
Now that we have the mass in kilograms, we can use the equation. We need to remember to give our answer to 1dp and state the correct units.

⍴ = 4.5 / 0.000146
⍴ = 30821.91781
⍴ = 30821.9 kg/m³

The Particle Model

Particles are the fundamental units that make up all substances. The substances can be in one of three different states; solids, liquids and gases.

The arrangements and energies of the particles will affect the density of each state of matter.

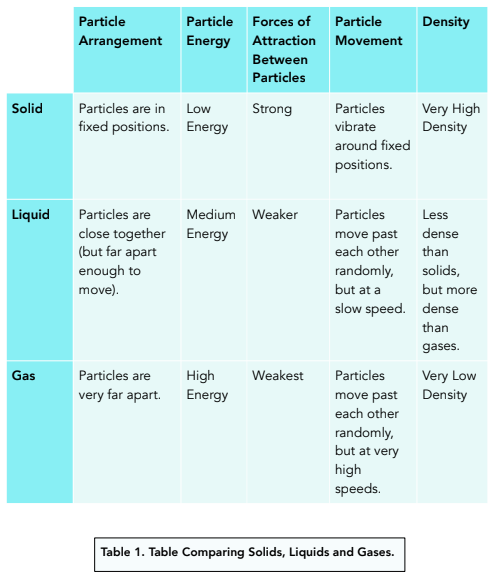
Comparing Solids, Liquids and Gases

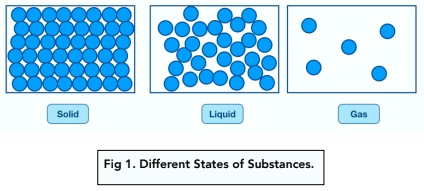
Below is a table showing the three states of matter and information about each one.

For AQA exams, you will be expected to be able to recognise and draw the diagrams shown below.

Investigating Densities

To measure the density of an object, we first need to work out the mass and volume. Then, we can use our equation density = mass x volume to get density.

For AQA exams, we need to be able to work out the density of solid objects and the densities of different liquids.

Method for a Regular Solid

1. Gather all the equipment. For this experiment, we will need a solid object, a top pan balance and a measuring ruler.
2. Measure the mass. Place the object onto the top pan balance and measure the mass of the object. Record the mass in grams.
3. Work out the volume using measurements. To measure the volume of a regular solid, you can use measurements and the appropriate formula. For example, the volume of a cube is width x length x height. For example, if you had a sphere, just use the formula V = 4/3 π r³. Record the mass in cm³.
4. Calculate density. Using the formula density = mass x volume, we can calculate the density. Previously, we measured the mass in grams (for accuracy). Now, we need to convert this mass into kilograms. Similarly, we recorded the volume in cm³ for accuracy, so we need to convert it into m³.

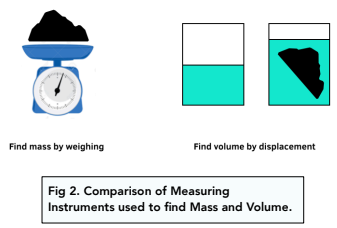
Method for an Irregular Solid

1. Gather all the equipment. For this experiment, we will need a solid object, a top pan balance and a measuring beaker filled with water.
2. Measure the mass. Place the object onto the top pan balance and measure the mass of the object. Record the mass in grams.
3. If the object is irregular, work out the volume using displacement. To measure the volume of an irregular solid, you can use displacement. Place the solid in a beaker of water, and the increase in water volume shows the volume of solid. This is shown in the diagram below.
4. Calculate density. Using the formula density = mass x volume, we can calculate the density.

Question: Adi is doing an experiment on density at school. He is finding the density of three items: a key, a piggy bank, and a jigsaw piece. Adi weighs each item, and then uses displacement to work out the volumes.

Work out the density of each of the three items.

1. Work out the volumes of each item

K= Key (5g), J=Jigsaw (7.5g), P=Piggybank (13g)

The empty beaker is 15cm³, and so when forming the equations, it is
important to subtract 15 from the volume.

By comparing 2 and 3, we can see that the pig weighs 13 cm³ more than
the jigsaw (because they both have a key and no other difference).
Therefore p = j + 13

1 p = j + 13
2 p + j = 21

We can re-arrange

1 p – j = 13
2 p + j = 21

And then subtract 1 from 2 (i.e. 2 minus 1)

2j = 8
j = 4

Plug back in j=4 to work out P

p – j = 13
p – 4 = 13
p = 17

Plug in j=4 to work out K

j + k = 11
4 + k = 11
k = 7

So overall:

p = 17
j = 4
k = 7

2. Calculate density using mass and volume

Density = Mass/Volume

Density of K: 5/7 = 0.7 g/cm³
Density of J: 7.5/4 = 1.9 g/cm³
Density of P: 13/17 = 0.8 g/cm³