Trajectories
Projectile Motion
- An object in projectile motion follows a path that can be divided into vertical and horizontal components
- Each component needs to be analyzed independently
- Here are some important terms to be familiar with and how to compute them:
- Time of flight: duration of the projectile’s time in the air
- Maximum height: the highest point the projectile reaches before momentarily stopping
- Range: the horizontal distance covered by the projectile
Trajectory Formula
- Projectile motion refers to the curved path followed by an object as it moves under the influence of gravity. In this discussion, we will explore the trajectory of projectile motion, its formula, derivation, and some examples.
Trajectory
Projectile motion is the movement of an object thrown into the air, following a curved path under the influence of gravity. Galileo was the first to observe this curved path, which is also known as the projectile’s trajectory. The trajectory is a form of ballistic motion, where gravity plays a significant role in determining the object’s path.
Trajectory Formula
The trajectory formula is used to calculate the force of gravity acting on an object during its flight. The trajectory is determined by the object’s vertical (y) and horizontal (x) position components.
When a projectile is launched with an initial velocity v0 at an angle θ from the horizontal plane, the vertical position of the object in terms of its horizontal position can be determined using this formula.
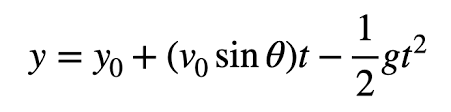
The formula for the trajectory is derived
y = vertical position of the object in meters
x = horizontal position of the object in meters
v0 = initial velocity of the object in meters per second
g = acceleration due to gravity, which is 9.80 m/s2
θ – initial angle from the horizontal plane in degrees or radians
Projectile Motion
Projectile motion is the type of motion where an object follows a symmetrical and parabolic path, known as its trajectory. It occurs when only one force is applied at the start of the trajectory, after which the object is solely influenced by gravity. In a previous section, we discussed the different components of an object in projectile motion. In this section, we will cover the fundamental equations associated with these components, assuming the initial positions of the projectile are null (i.e., x0=0 and y0=0).
What does Projectile Motion mean?
Projectile motion refers to the movement of an object along a symmetrical and parabolic path, called its trajectory. This type of motion occurs when an object experiences a single force at the beginning of its path, and from then on, the only force acting on the object is gravity.
What are the Essential Elements of Projectile Motion?
To solve problems related to projectile motion, it is important to remember the following essential elements:
- Initial launch angle, θ
- Initial velocity, u
- Time of flight, T
- Acceleration, a
- Horizontal velocity, vx
- Vertical velocity, vy
- Displacement, d
- Maximum height, H
- Range, R
A trajectory is the path that a projectile takes through the air. It is influenced by factors such as gravity, air resistance, and initial velocity.
The key equations for calculating trajectories include the range equation, the maximum height equation, and the time of flight equation. These equations take into account factors such as initial velocity, angle of launch, and acceleration due to gravity.
To calculate the range of a projectile, you can use the range equation: R = (v0^2 * sin(2θ))/g, where R is the range, v0 is the initial velocity, θ is the angle of launch, and g is the acceleration due to gravity.
A horizontal trajectory is one in which the projectile travels parallel to the ground, while a vertical trajectory is one in which the projectile travels directly up or down.
Air resistance can affect trajectories by slowing down the projectile and changing its direction. The effect of air resistance is greater for objects with a larger surface area and a lower mass.
Trajectories are used in a variety of real-world applications, such as ballistics, aerospace engineering, and sports. For example, trajectories are used to design missiles, rockets, and satellites, and to calculate the trajectory of a golf ball or a basketball.
To improve your understanding of trajectories in A-Level Physics, you can practice solving problems using the key equations, watch online tutorials, and read textbooks and study guides. You can also seek help from your teachers or tutors if you need additional support.





Still got a question? Leave a comment
Leave a comment